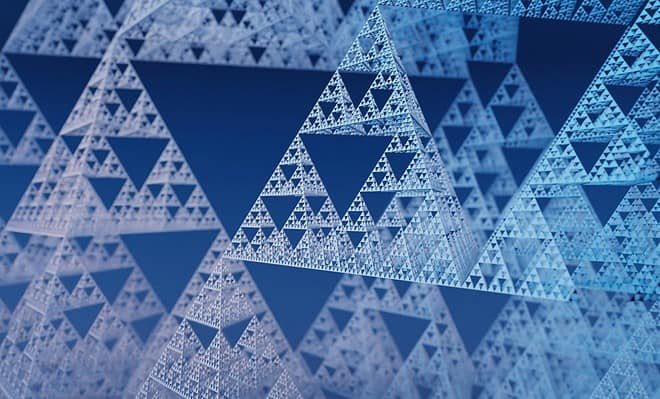
In April 2024, Sendker et al. released a paper in the scientific journal Nature about the finding that a bacterial molecule, citrate synthase, forms Sierpinski triangles. A Sierpinski triangle is a famous example of triangles composed of triangles, such that if you observe the inner triangles, those too are composed of even smaller triangles. For the theoretical concept, no matter how far you zoom in or out on a Sierpinski triangle, or any other fractal, the self-similarity persists. However, in the natural world not contained within a math proof, common examples of non-Sierpinski triangle fractals exist in outer space, coastlines (Karl Wierstrass’s jagged line), fern leaves, and the alveoli of the human lung. These lack the infinite ability to change resolution and still observe the recursive pattern. Citrate synthase’s fractal structure is another example of a non-infinite fractal in nature that supports Chaos Theory.

Chaos Theory

Unlike the comfortable and concrete explanations of scientific concepts like gravity, electrical conductivity, and stoichiometry, Chaos Theory addresses the unpredictable and disordered states of the natural world and the constant change observed within it. This, in turn, results in the existence of finite fractals we observe.

The concept of “chaos” suggests an oscillation between order and disorder. Theoretical predictions that may not be possible to realize depend on accounting for unknowable variables. This is relevant in complex systems, such as those observed in the natural world (as opposed to simulated), where due to unknowable variables, some degree of error will always exist, resulting in imperfect predictions by mathematical models.

“Real life isn’t a series of interconnected events occurring one after another like beads strung on a necklace. Life is actually a series of encounters in which one event may change those that follow in a wholly unpredictable, even devastating way.”
― Michael Crichton, Jurassic Park

Relevance To Biology

Therefore, the existence of a fractal conformation of a biological molecule supports the recursive properties of protein conformation previously suggested. However, this is not biologically active and bolsters the idea that disorder is biologically active, whereas order is biologically inactive.

Citrate synthase is part of the TCA or Krebs cycle. This metabolic process exists in almost all life on Earth, including a cyanobacterium Synechococcus elongatus (“held as an elongated grain”), the source of the citrate synthase in the paper. Cyanobacteria have four different variants of this metabolic cycle, which support their ability to metabolize self-derived photosynthetic products. The pH of the location of metabolism within cells is not the same as water, the solvent used in the study. Cyanobacterial metabolism requires slightly more basic than neutral pH for optimal activity.

Why Does pH Matter?

But why does that matter? Why is pH important to consider? The pH is a measurement of the acidity or basicness of a solvent, though more importantly, this refers to the concentration of free hydrogen protons within a solution. Macromolecules, such as enzymes like citrate synthase, form shapes based on energetic favorability within their environment, such as interactions with hydrogen or hydroxide in solution.

The authors recognize this and in the paper, they share, “Indeed, an increase in the pH from 7.5 to 9 led to complete disassembly of the structure into hexamers.” This means that, while fascinating, this structure is unlikely to be functional and instead shows unique characteristics of a non-natural conformation state change. Unlike other examples of fractals in nature, citrate synthase forming Sierpinski triangles is unlike other biological examples provided, as these exist in functional states, making the conformation of citrate synthase akin to fractal lattice structures found in crystals.

Conclusion

While we do not yet understand the greater implications of this discovery, we understand it offers yet another way fractals exist outside of mathematical proofs. Although we do not yet understand the full biological implications, I hope the reader can take a moment to appreciate how finding self-similar patterns from molecules to outer space presents an example of the astounding mystery and awe existing in this universe also exists within.